Stable

 The natural logarithm, ln - based on the number e - is the tool

of choice for those working on time and growth problems. Ever

wonder why.

Looking up the e article on the French Wikipedia, I was struck by the

fact that graph below is how the number is actually defined. Not just an

interesting factoid about e but its precise definition. Using the function 1/x:

Had to think this thing out for a while...Did look suspicious e is an irrational

number ( impossible to express as a fraction). Indeed. it is a transcendental number

and cannot be expressed as the root to any equation. (In contrast, the square root

of 2 is irrational but not transcendental because it is a root). It is a never ending

sequence of numbers 2.718281828459045235360...

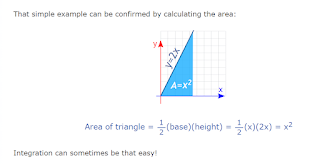
Now integration in Calculus is used to define an area under a curve. Math is Fun introduces

the notion of integration with this fun illustration:

Now that is clear enough, and the area in question is something fixed.

But the area under the curve with e - 1 as a side had me worried. But that is the

cleverness of the whole thing, isn't is. Whatever unruliness e creates in the calculation

is compensated by that of the curve of the function. Our area is a stable 1!!

Our formula is thus one for a definite integral, between 1 and e, which subtracts

the portion between 0 and 1, which is 0, and ignores everything

that comes later then e:

                                                               

Math is Fun suggests memorizing e as:

2.7  1828  1828  45  90  45...